Historic Sand Rock Farm Rake Crown Moulding

Historic Sand Rock Farm Bed & Breakfast in Aptos California, built in the 1880's, is one of the original family wineries in the Monterey Bay Area. There's a wine cellar on the grounds, 40'x60', where the aging roof had been removed and used as part of the  Sand Rock Farm wedding facilities.  It was a great place to have my daughter's wedding this past weekend.

My wife, my daughter Sabrina, her new husband Dardy, his best man Alex and my son Erik dancing the night away in the wine cellar. 

However, I'm concerned about it remaining historic over the coming years. The current owner bought   Sand Rock Farm in 1999 when it was in despair. She rejuvenated the property into a Bed & Breakfast and wedding facility. Sand Rock Farm will have a new owner in the next couple of months and hopefully they will restore the original buildings roof and eave rake crown moulding detail, so it remains historic. Rather than remove the eave and rake crown moulding and replace it with metal rain gutters.

Gary Katz at ThisIsCarpentry has an article on Get Your House Right: Architectural Elements to Use & Avoid . The article goes thru the steps of drawing out the different profiles for rake crown moulding. The frieze trim on this house seems a little big, but I wouldn't change the size of the frieze when restoring the trim moulding. 

If I were to replace the rake crown moulding on this house, I would cut a piece of the existing rake crown moulding and send it to a custom mill in the area. The reproduction rake crown moulding would have two different profiles on this house. One for the rake crown going up the gable ends and one for the horizontal crown moulding. I would have it milled out of redwood to make sure it lasted another 100 years.

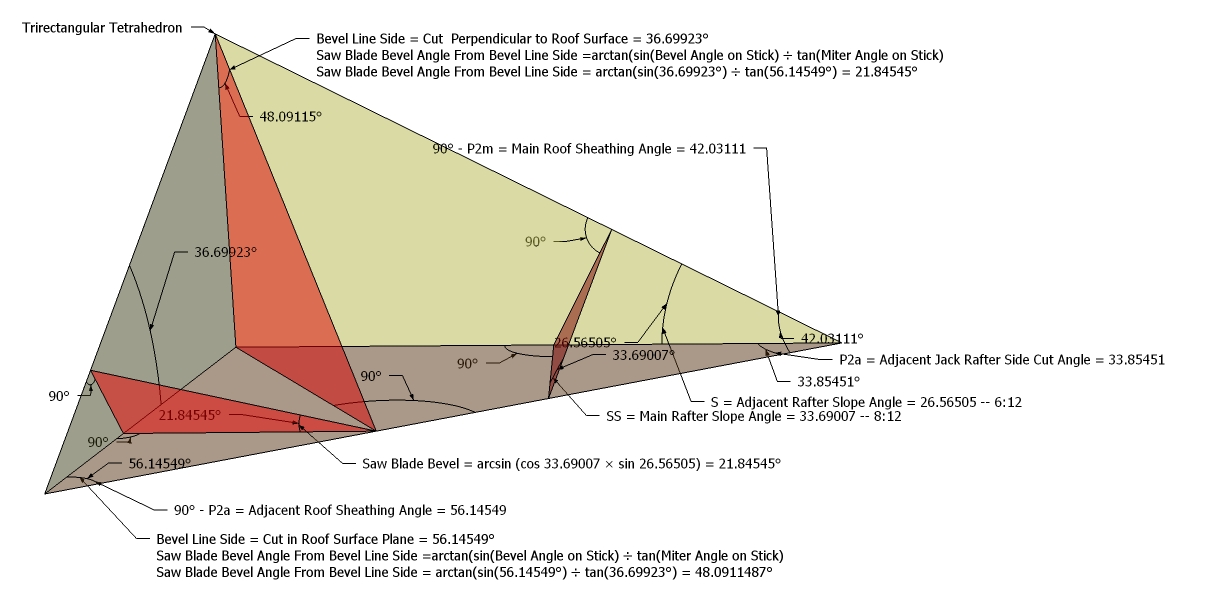
The actual rake crown moulding compound miter cuts on this house are pretty easy. You could use my Android or Apple Crown Moulding tools apps to cut the rake crown moulding laying flat in a compound miter saw. Or use geometry to draw out the compound miter cuts and cut the rake crown moulding nested. I think the roof pitch on this house is a 9:12 pitch ,36.86989°. For the horizontal to rake  crown  miter cut you would use half of the slope angle, 36.86989° ÷ 2 = 18.734948°.  The rake crown moulding cuts at the peak of the roof are the same angle as the roof slope angle.

For cutting the nested rake crown moulding miter cuts at the gable returns.

1. For the Rake crown moulding going up the rake use the roof slope angle with the saw blade bevel angle set to 45°. The top edge will be nested against the miter saw fence.
2. For the horizontal crown moulding use a miter angle of 45° with the saw blade bevel angle set to 0°. The bottom edge of the crown moulding will be nested against the fence.

If you've come to this page on Sand Rock Farm by  searching the web for wedding planners or consultants you should contact Lynn & Kristen. There are many pieces to planning a wedding ceremony and Lynn & Kristen made sure the wedding was  executed flawlessly.   

Lynn Myers-Galster
Professional Wedding Consultant
www.dukeandpearl.com

Perfect setting for the wedding ceremony under this 500 year old redwood tree that's as  straight as an arrow.

Trigonometric Formulas Geometrically

Trigonometry comes from the Greek trigōnon ("triangle") and metron ("measurement").

Geometry comes from the Greek geo ("earth") and metron ("measurement"). 

The Greek mathematicians Euclid and Archimedes in the 3rd century BC were the first to prove trigonometric formulas geometrically. You can find the values of sine, cosine and tangent functions, by using a unit circle with a radius = 1. This article will explain how to develop Hip -Valley Rafter Roof Ratios using  Trigonometric Formulas geometrically drawn using Tetrahedrons or Trirectangular Tetrahedrons for the Trigonometric Ratios we use in roof framing. The tetrahedron unfolded is the same as the roof framing kernel. The trirectangular tetrahedron unfolded is a tetrahedron slice from a compound joint. 

The "Unit Circle" is a circle with a radius of 1.

For a triangle with an angle θ, the trigonometric ratios functions are calculated this way:

Sine Function:	sin(θ) = Opposite / Hypotenuse
Cosine Function:	cos(θ) = Adjacent / Hypotenuse
Tangent Function:	tan(θ) = Opposite / Adjacent

For a triangle with an angle θ, the trigonometric ratios functions are calculated this way for roof framing:

Sine Function:	sin(θ) = Rafter Rise / Rafter Length
Cosine Function:	cos(θ) = Rafter Run / Rafter Length
Tangent Function:	tan(θ) = Rafter Rise / Rafter Run

Because the radius is 1, you can directly measure sine, cosine and tangent.

Because the radius is 1, you can also directly measure cosecant, secant and cotangent.

Because the radius is 1, you can also directly measure the Trigonometric Ratios of an polygon. An Octagon in this example.

Basic Roof Framing Tetrahedron unfolded.

D Angle = Plan Angle

A Angle = Roof Slope Angle

C Angle = Hip Rafter Slope Angle

E Angle = Jack Rafter Side Cut Angle

B Angle = Hip Rafter Backing Angle

In this example the triangle in blue has the following properties:

Roof Slope Angle = ß  = 33.69007°

Plan Angle = α = 45.00°

Radius = 1

The altitude of the triangle can be found by multiplying the sine of the triangle by the cosine of the triangle. 

Altitude of Triangle =  sin ß × cos ß

sin ß = 0.5547

cos ß  = 0.8321

Altitude of Triangle =  sin ß × cos ß = 0.5547 × 0.8321 = 0.4615

In this example the triangle in blue has the following properties:

Hip Rafter Slope Angle = ß  = 25.23940°

Plan Angle = α = 45.00°

Radius = 1

 Again, the altitude of the triangle can be found by multiplying the sine of the triangle by the cosine of the triangle. 

Altitude of Triangle =  sin ß × cos ß

sin ß =0.4264

cos ß  =  0.9045 

Altitude of Triangle =  sin ß × cos ß = 0.4264 × 0.9045 = 0.3857

If were studying tetrahedrons for roof framing trigonometric ratios we could also use following trigonometric ratios to find the base length of the plan angle triangle or the height of the plan angle triangle.

cos α × cos ß =  base length of the plan angle triangle

cos α × cos ß = 0.6396

0.7071 × 0.9045 = 0.6396

sin α × cos ß = height of the plan angle triangle

sin α × cos ß = 0.6396

0.7071 × 0.9045 =0.6396

Since the Altitude of Triangle =  sin ß × cos ß
we can use the following Trigonometric Ratios to write an formula for the hip rafter backing angle when the plan angle is equal to 45.00°.

Hip Rafter Backing Angle = 
arctan((sin ß × cos ß) ÷ cos ß) =
arctan((sin ß × cos ß) ÷ cos ß) =
arctan(sin ß)  =

arctan(sin 25.23940°) = 23.09347°
arctan(sin(Hip Rafter Slope Angle)) = Hip Rafter Backing Angle

When the plan angle is not equal to 45.00° you need to use the tan of the plan angle in the trigonometric formula for the hip rafter backing angle.

Hip Slope Angle = ß  =  27.50055°
Plan Angle = α = 51.34019°
Radius = 1

Hip Rafter Backing Angle = 
arctan((sin ß × cos ß) ÷ (cos ß × tan α)) =
arctan((sin ß × cos ß) ÷ (cos ß × tan α)) =
arctan(sin ß ÷ tan α) =
arctan(sin 27.50055° ÷ tan 51.34019) = 20.27452°
arctan(sin Hip Rafter Slope Angle ÷ tan Plan Angle)= Hip Rafter Backing Angle

In this example you can find the trigonometric ratios to write a formula for the Hip Rafter Slope Angle, angle C in the unfolded tetrahedron.  You have to extend the base of triangle A, Rafter Slope Angle Triangle, so the Hypotenuse (Rafter Length) is equal to 1. So, you can dimension the correct length of the cosine of the Rafter Slope Angle.

Angle C = arccos( cos( A ) ÷ cos ( B ))

or

Hip Rafter Slope Angle = arccos( cos( Roof Slope Angle ) ÷ cos (Hip Rafter Backing Angle ))

cos A = 0.83205

cos B = 0.93804

cos C = ( 0.83205 ÷ 0.93804)

cos C = ( 0.88701)

Angle C = arccos( 0.83205 ÷ 0.93804) =27.50055

Angle C = arccos(0.88701) =27.50055

It's useful to print out the Unit Circle Trigonometric Functions-Ratios for the triangles you're studying, when you're trying to write roof framing formulas.  This way you can try different combinations of the trigonometric ratios using multiplication or division. 

Example using   multiplication of the cosine of D and the values of angle A:

0.62470 × 0.83205 = 0.51978 ...no match

0.62470 ×  0.55470 = 0.34652 ...matches the sin of the angle B

0.62470 ×  0.66667 = 0.41647 ...no match

From these matches we can write

Angle B = arcsin(cos(D) × sin(A))

Hip Rafter Backing Angle = arcsin(cos(Plan Angle) × sin(Roof Slope Angle)) 

D Angle = 51.34019 = Plan Angle

cos = 0.62470

sin = 0.78087

tan = 1.24999

A Angle = 33.69007 = Roof Slope Angle

cos = 0.83205

sin = 0.55470

tan = 0.66667

C Angle = 27.50055 = Hip Rafter Slope Angle

cos = 0.88701

sin = 0.46176

tan = 0.52058

E Angle = 33.64933 = Jack Rafter Side Cut Angle

cos = 0.83244

sin = 0.55411

tan = 0.66564

B Angle = 20.27452 = Hip Rafter Backing Angle

cos = 0.93804

sin = 0.34652

tan = 0.36941

Here's an example for writing an trigonometric formula for the tetrahedron angle E, jack rafter side cut angle.

Angle E = arctan(cos(A) ÷ tan(D))

Angle E = arctan(0.83205 ÷ 1.24999)

Angle E = arctan(0.66564) = 33.64933 

Jack Rafter Side Cut Angle = arctan(cos(Roof Slope Angle) ÷ tan( Plan Angle))

Here's an example of using the values of tan, cosine and sine of a tetrahedron to write a trigonometric for the Angle D in the tetrahedron. (D = Plan Angle)

Angle D = arccos(sin(E) ÷ cos(C))

Angle D = arccos(0.55411 ÷ 0.88701)

Angle D = arccos(0.62470) = 51.34019

Plan Angle = arccos(sin(Jack Rafter Side Cut Angle) ÷ cos( Hip Rafter Slope Angle))

Layover Cuts #2

Purlin Rafters, Roof Sheathing or Dormer Square Tail Fascia Layover Cuts
(Cutting Roof Sheathing with an Edge Bevel)

I was asked to elaborate on roof framing layover cuts. Using the same valley sleeper angle to cut the valley sleeper bevel angle and the layover roof sheathing seems wrong, but it is correct.

Always make some type of test model while you're studying roof framing angles.

Layover cut with an irregular valley. 6:12 & 8:12 roof pitches.

Layover cut with an regular valley. 8:12 roof pitch. 

Trirectangular Tetrahedron example for irregular hip roof layover cuts.

C5m Main Hip Rafter Backing Angle = 26.34404
C5a Adjacent Hip Rafter Backing Angle = 15.56481

P2m = Main Jack Rafter Side Cut Angle = 47.96889
90° - P2m = Main Roof Sheathing Angle = 42.03111
P2a = Adjacent Jack Rafter Side Cut Angle = 33.85451
90° - P2a = Adjacent Roof Sheathing Angle = 56.14549

Bevel Angle = arctan(tan(C5m + C5a) × sin(90° - P2a))
Bevel Angle = arctan(tan(26.34404 + 15.56481) × sin(90° - 33.85451°))
Bevel Angle = arctan(tan(41.90885°) × sin(56.14549)) = 36.69923°

Bevel Line Side = Cut Perpendicular to Roof Surface = 36.69923°
Saw Blade Bevel Angle From Bevel Line Side =arctan(sin(Bevel Angle on Stick) ÷ tan(Miter Angle on Stick)
Saw Blade Bevel Angle From Bevel Line Side = arctan(sin(36.69923°) ÷ tan(56.14549°) = 21.84545°

Bevel Line Side = Cut in Roof Surface Plane = 56.14549°
Saw Blade Bevel Angle From Bevel Line Side =arctan(sin(Bevel Angle on Stick) ÷ tan(Miter Angle on Stick)
Saw Blade Bevel Angle From Bevel Line Side = arctan(sin(56.14549°) ÷ tan(36.69923°) = 48.0911487°

Napa EarthQuake Structural

Here's some pictures of the buildings I visited on August 31 2014. 7 days after the 6.0 Earthquake in Napa California. The structural damage I viewed suggested that it was a unreinforced masonry - brick tension tie failure, or lack of tension ties. Unreinforced masonry was banned in California in 1930, due to its vulnerability during earthquakes. Downtown Napa is open for business and the earthquake damage is a new tourist attraction. Walking around Napa, it looks like there's only about 1% of the buildings in Napa there were damaged by the earthquake. The Historic Napa Mill and RiverFront Inn looked like it had no exterior damage to the 3 story building.

Extreme Danger

Stay Back 100 Feet

This National Disaster Team didn't read the Extreme Danger sign. 

So, what is in those two trucks? Or are they there just for advertising?

I was in this building 6 years ago. I remember seeing the tension ties running thru the building. The main damage to this building was the front of the building where there are no tension ties.

Here's the famous dangling roof that was part of a recent retrofit. Apparently the tubular steel with rods 16" O.C. should had the rods protrude thru the exterior wall with plate washers. It probably needed an steel uplift post at the corner as well.

This church with the exterior parapet wall detached from the roof framing needed some type of wall to roof connection. This is a lot of weight leaning towards the street. It needs some raker bracing.

Wood frame structure

Wood is one of the best materials for earthquake-resistant construction since it is lightweight and more flexible than masonry.  This Victorian house and 100's more are right across the street from the church with the leaning rake wall. These Victorian homes didn't appear to have any exterior wall framing damage from the earthquake. I had forgotten how many Victorian houses are in downtown Napa. 

The GoodMan Library, historical society, needed bigger tension ties to hold the exterior brick-stone walls together in an earthquake.Again it should of had tension ties with plate washers on the outside of the building.

This church had stain glass windows blown out in the earthquake. However, the exterior framing looked pretty good.

I stayed in the Church Hill Manor bed & breakfast Inn 6 years ago and it didn't appear to have any damage from the earthquake.

The United States Post Office in Napa definitely needs some repair. Again it could've used some tension ties with plate washers to prevent this crack in the exterior wall of the building. Right across the street from the post office is a newer building with all glass exterior walls and some brick veneer. This building looked completely unaffected by the earthquake.

The castle, Napa State Hospital -- Asylum for the Insane, was torn down after world war II. However, it represents the love affair with building with bricks in the 19th century. I researched to see why it was torn down, but never found an answer. This brick building was doomed from the time the first brick was laid.

Link to all of my pictures of the Napa Earthquake.